Ampere’s Circuital Law is one of the basic elements that predict the relation between electric currents and magnetic fields. For the students of physics, this law is important not only in higher studies but also while preparing for any type of competitive examination. Let us explore Ampere’s circuital law, its proof, and its applications in the following article.
Who Was André-Marie Ampère?
André-Marie Ampère was a scientist who experimented with how forces act upon wires carrying electric currents. He did this in the late 1820s, around the same time as Faraday’s work on Faraday’s Law. Neither Faraday nor Ampère realized that Maxwell would eventually combine their work four years later.
What is Ampere’s Circuital Law?
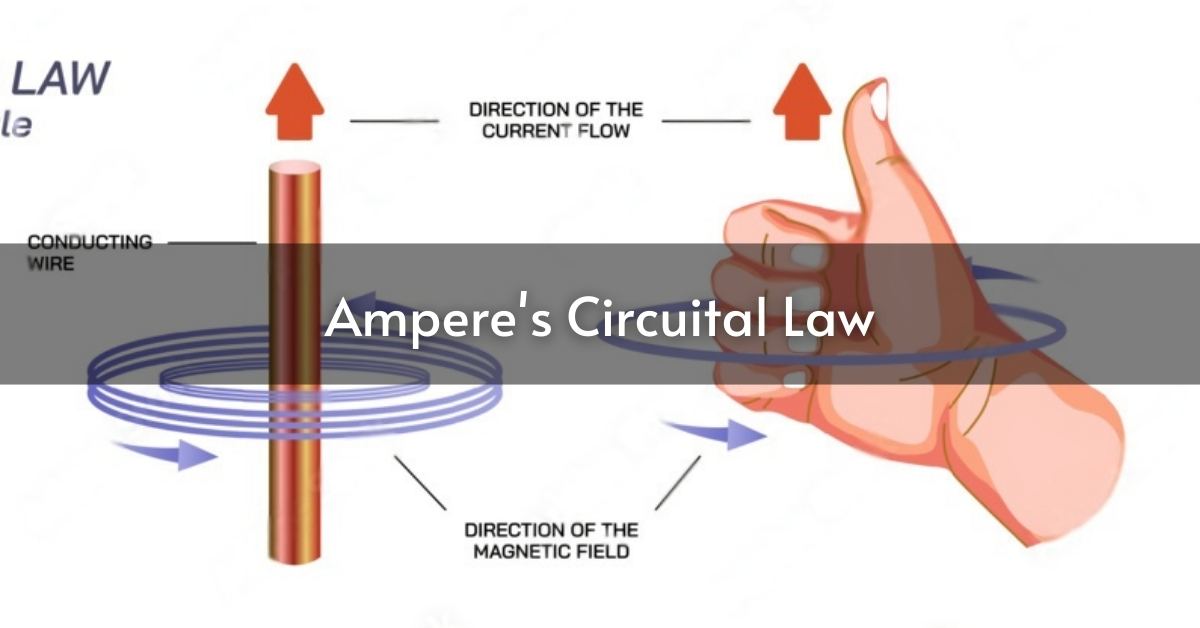
Ampere’s Circuital Law states that the line integral of the magnetic field around a closed loop is proportional to the total electric current flowing through the loop. Mathematically, it is written as:
∮ B · dl = μ₀ Inet
Here, B is the magnetic field, dl is a differential length element of the closed loop, μ₀ is the permeability of free space, and Inet is the total current enclosed by the loop. This relationship is critical to performing computations on the magnetic field around current-carrying conductors.
Understanding the Components of the Law
There are many parts to the law, and understanding those parts is very important to apply this law:
- Magnetic Field (B) : The magnetic field caused due to electric currents.
- ∮ B • dl: Magnetic field line integral The magnetic field around a closed path is an integration.
- Total Current (Inet): The vector sum of currents passing through the loop.
- Magnetic Constant or Permeability of Free Space (μ₀): It is the constant through which magnetic field strength and current are related.
Proof of Ampere’s Circuital Law
To understand the idea of Ampere’s Circuital Law, proving the law is important. The proof deals with the straight wire which carries some current and the application of the law to a circular path around it.
Case 1: Uniform Coil
Now take up a uniform coil carrying some current I. The magnetic field owing to this coil is given at distance r from the wire as:
B = μ₀ I / (2πr)
When the field is integrated around a closed loop, we write the total line integral as
∮ B • dl = B ∮ dl = μ₀ I
Here ∮ dl represents the circumference of the loop and this leads to the fact that the line integral of the magnetic field along the closed loop is just μ₀ times the total enclosed current.
Case 2: Irregular Coil
For a tortuous coil, where the radius may be a function of position along the coil, the integral is more complicated. However, applying the same argument, one can prove that:
∮ B • dl = μ₀ Inet
This shows that it is true for any shape of the loop.
Applications of Ampere’s Circuital Law
Ampere’s Circuital Law has a wide application in physics and engineering in the design of electrical devices and analysis of magnetic fields.
- Calculation of Magnetic Fields: It finds extreme applications in calculating the magnetic field that is produced by long straight wires, solenoids, and toroid’s.
- Electromagnetic Devices: The law is important for the design of inductors, transformers, and electric motors.
- Magneto Field Mapping: Ampere’s Circuital law makes it possible to visualize and map in space the magnetic fields that have a simple geometrical structure.
Ambiguities and Sign Conventions
There are a few unclear points in these definitions that need to be sorted out, and a convention needs to be chosen.
To summarize, then three terms in Stokes’ Theorem involve sign ambiguities: the line integral ∮C can be integrated either clockwise or counterclockwise around the loop; the vector area dS points in one of two possible directions normal to the surface; and Ienc is the net current passing through the surface S. This net current is the current passing in one direction minus the current passing in the other, but either direction can be declared positive.
To resolve this ambiguity, apply the right-hand rule: if your right-hand palm is in the direction toward the region of integration and your index finger is parallel to the line integral direction, then your thumb points in the direction in which to take for the vector area dS. You can assume to count current as positive if it flows in the same direction as dS. You can also apply the right-hand grip rule to determine the signs:.
There is also no unique ambient surface S whose boundary is the curve C. Consider the soap film bounded by a wire loop: if you blow on it, it may change shape. Which surface do you take? The answer depends on the possibility-or lack thereof-that the loop lies in a single plane.
The good news is that it doesn’t matter: in the magnetostatic case, the current density is solenoidal (as explained in the next section), so the flux through any surface with boundary C, as long as the sign convention is the same, will be the same. In practice you usually pick the most convenient surface to integrate over.
Free Current vs. Bound Current
Free current is the electric current that you are familiar with in simple cases: flow within a wire or a battery. However, bound current occurs in materials that can be magnetized or polarized; actually all materials can to some extent.
When such material is magnetized (put it in an external magnetic field), then the electrons stay bound to their atoms but circulate around within such an arrangement so that they generate a small current. If all those small currents generated at each and every atom are added up, they sum up into one much larger, continuous current whose flow encircles the magnetized material. This one is termed as magnetization current or JM which falls under the category of bound current.
The other bound current is that from the bound charges. You can cause a separation of positive and negative charges within some tiny distances along with an application of an electric field, thus altering the polarization of a material. With the movement of the bound charges created, there is another bound current known as the polarization current (JP).
Where, Jf is free or conduction current density.
Because all currents are the same at the microscopic level anyway, it is often convenient in practice to distinguish between bound and free currents. Bound currents typically arise from atomic phenomena, so that more manageable theories that hold at the macroscopic scale often suffice. As a result, Ampere’s law, which typically contains free, magnetization, and polarization currents, reduces in most cases to the free current and magnetic field (H).
Understanding the Amperian Loop
The Amperian loop is an imaginary closed path in applying Ampere’s Circuital Law. The reason it is special is that the magnetic field has to be constant or zero along the path for the law to properly apply. The shape of the loop can vary but must enclose the current effectively.
Conclusion
Ampere’s Circuital Law is an integral part of electromagnetism, which connects electricity and magnetism. The ability to know the principles, proof, and applications of this law will help students explore electromagnetic theory and how it exists around them. The law amplifies knowledge of the magnetic field, thereby proving to be very crucial in the design and working of many electric devices.
Frequently Asked Questions
What is the significance of Ampere’s Circuital Law?
It describes how electric currents are related to magnetic fields, which is the most basic concept in electromagnetism.
How does one determine the Amperian loop?
The choice of the loop makes the calculation straightforward; one takes an American loop such that the magnetic field is constant or zero.
Can one apply Ampere’s Circuital Law on any shape of loop?
Yes, but calculations will vary with symmetry and the configuration of the loop.
Read More – 3-phase induction motor, Electrical, How Electric Motors Work